40 Essential and Uncommon Mathematical Symbols: A Quick Guide

Mathematics, often called the universal language, is rooted in symbols that simplify complex ideas, making them easier to express and understand. From basic arithmetic to advanced calculus, symbols form the foundation of mathematical notation.

They allow us to represent operations, equations, and relationships concisely. Whether you’re a student just beginning to explore algebra or a seasoned professional diving deep into calculus, mastering these symbols is crucial for success.

This guide covers 40 key mathematical symbols—common and lesser-known—providing clear explanations of their usage and examples to help you understand how they apply to real-world problems.

From the simple plus sign to the more intricate tensor product, each symbol holds significant value in mathematical language.

Explore this detailed list and enhance your mathematical fluency, gaining a stronger grasp of how these symbols are applied across different operations.

Common Mathematical Symbols

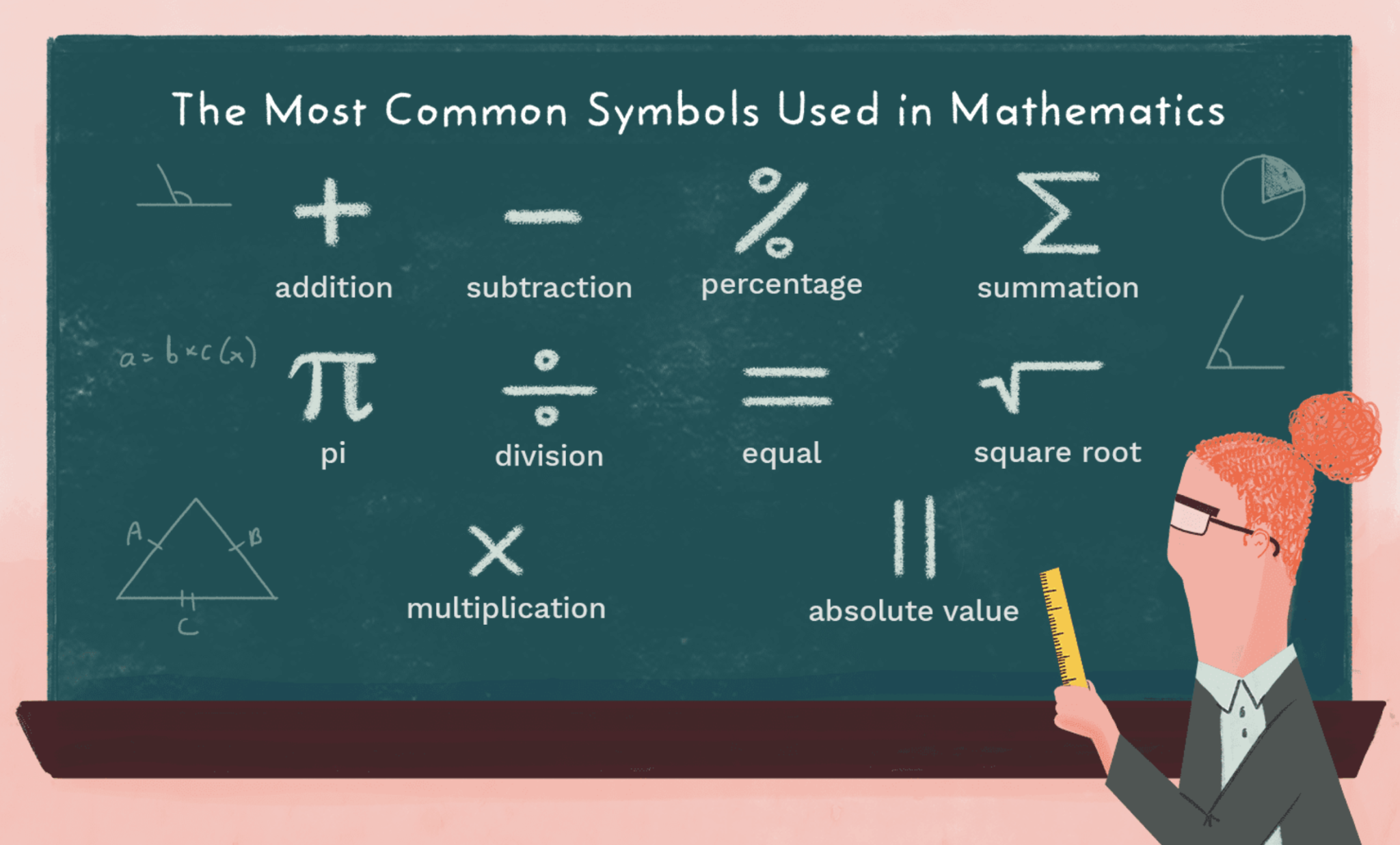
1. Symbol: ‘+’
Name: Plus Sign
Why it is Used: The plus sign is used for addition, a fundamental mathematical operation. It indicates that two or more numbers or values are being combined to calculate their total. Addition is widely used in everyday arithmetic and in more complex mathematical operations.
Example:

• Basic operation: 5 + 3 = 8
• Adding multiple values: 10 + 4 + 6 = 20

2. Symbol: ‘−’
Name: Minus Sign
Why it is Used: The minus sign represents subtraction, a basic operation in which one value is taken away from another. It is essential for solving problems in arithmetic, algebra, and many other branches of mathematics. Subtraction is also used to find the difference between numbers.
Example:

• Basic operation: 9 − 4 = 5
• With negative numbers: 7 − 10 = −3

3. Symbol: ‘×’
Name: Multiplication Sign
Why it is Used: The multiplication sign signifies multiplying two or more numbers together. It is an essential operation in arithmetic, algebra, and many areas of mathematics and science. Multiplication is often described as repeated addition.
Example:

• Basic multiplication: 4 × 3 = 12
• With decimals: 5.5 × 2 = 11

4. Symbol: ‘÷’
Name: Division Sign
Why it is Used: The division sign represents the operation of dividing one number by another. It is the process of determining how many times one number is contained within another, and it’s the inverse of multiplication. Division is widely used in arithmetic, algebra, and fractions.
Example:

• Basic division: 12 ÷ 4 = 3
• With decimals: 10 ÷ 2.5 = 4

5. Symbol: ‘=’
Name: Equal Sign
Why it is Used: The equal sign indicates equality between two expressions. It shows that the values or quantities on both sides of the equation are the same. The equal sign is a crucial part of writing mathematical equations and solving problems in various branches of mathematics, including algebra, geometry, and calculus.
Example:

• Basic equation: 5 + 2 = 7
• Complex equation: 3x + 4 = 19

6. Symbol: ‘≠’
Name: Not Equal Sign
Why it is Used: The not equal sign is used to show that two expressions or values are not the same. It is often used in comparisons, inequalities, and when stating that a solution does not satisfy a given equation.
Example:

• Basic inequality: 5 ≠ 4
• With variables: x ≠ 7

7. Symbol: ‘<‘
Name: Less Than Sign
Why it is Used: The less-than sign compares two numbers or expressions, indicating that the value on the left is smaller than the value on the right. It is widely used in inequalities, value comparison, and number theory.
Example:

• Comparison: 3 < 5
• With variables: x < 10

8. Symbol: ‘>’
Name: Greater Than Sign
Why it is Used: The greater than sign indicates that the value on the left is larger than the value on the right. It is the opposite of the less than sign and is frequently used in inequalities and comparisons.
Example:

• Comparison: 8 > 6
• With variables: y > 2

9. Symbol: ‘≤’
Name: Less Than or Equal To Sign
Why it is Used: The less than or equal to sign shows that a number or expression is either less than or equal to another. It’s a crucial symbol in inequalities and is commonly used in solving mathematical problems where a range of values is possible.
Example:

• Inequality: x ≤ 5
• With expressions: 2x + 3 ≤ 9

10. Symbol: ‘≥’
Name: Greater Than or Equal To Sign
Why it is Used: The greater than or equal to sign expresses that a value is either greater than or equal to another value. It is used in mathematics for inequalities and boundary conditions.
Example:

• Inequality: y ≥ 4
• With expressions: 3y − 2 ≥ 5

11. Symbol: ‘%’
Name: Percent
Why it is Used: The percent symbol represents a part per hundred and is used to express proportions, comparisons, and changes. Percentages are commonly used in finance, statistics, and everyday calculations.
Example:

• Simple percentage: 50%50\%50% of 100 = 50
• Change in percentage: A 20% increase from 50 is 50+(50×0.20)=6050 + (50 × 0.20) = 6050+(50×0.20)=60

12. Symbol: ‘√’
Name: Square Root
Why it is Used: The square root symbol is used to denote the number that, when multiplied by itself, gives the original value. It is essential in geometry, algebra, and number theory, particularly when solving quadratic equations.
Example:

• Basic square root: √16 = 4
• With decimals: √2 = 1.414

13. Symbol: ‘∑’
Name: Sigma (Summation)
Why it is Used: The summation symbol represents the sum of a series of numbers or terms. It is used extensively in calculus, statistics, and discrete mathematics to compute totals or to find sums over a set range.

Example: Summing integers: ∑ᵢ⁵; i=1+2+3+4+5=15

14. Symbol: ‘π’ 
Name: Pi
Why it is Used: Pi is a mathematical constant representing the ratio of a circle’s circumference to its diameter. It is used extensively in geometry, trigonometry, and calculus, especially in formulas involving circles.
Example:

• Circumference of a circle: C = 2πr
• Area of a circle: A = πr²

15. Symbol: ‘∞’
Name: Infinity
Why it is Used: The infinity symbol is used to represent an unbounded quantity, something that has no limits or end. It is used in calculus, set theory, and many branches of mathematics to describe endless processes or quantities.

Example: Infinite sum: ∑∞ n=1;1/n² =π²/6

16. Symbol: ‘!’
Name: Factorial
Why it is Used: The factorial symbol represents the product of all positive integers up to a specified number. It is commonly used in combinatorics, probability, and algebra to calculate permutations and combinations.

Example: Basic factorial: 5!=5 × 4 × 3 × 2 × 1 = 120

17. Symbol: ‘∫’
Name: Integral
Why it is Used: The integral sign is used in calculus to represent the integral of a function, which can be thought of as the area under a curve. It is fundamental in calculating areas, volumes, and other quantities in mathematics and physics.

Example: ∫ x² dx = x³/3 C

18. Symbol: ‘∂’
Name: Partial Derivative
Why it is Used: The partial derivative symbol represents the derivative of a function with respect to one variable while holding the others constant. It is widely used in multivariable calculus, differential equations, and physics.

Example: ∂/∂x = (x² + y²) = 2x

19. Symbol: ‘|x|’
Name: Absolute Value
Why it is Used: The absolute value symbol represents the distance of a number from zero on a number line, disregarding its sign. It is used in various mathematical disciplines, including algebra and complex numbers.
Example:

• Basic absolute value: |−5| = 5
• In an equation: |x − 3| = 7

20. Symbol: ‘≈’
Name: Approximately Equal
Why it is Used: The approximately equal symbol is used to show that two values are close to each other, but not exactly the same. It is often used in rounding numbers, estimations, and limits.
Example:

• Approximation: √2 = 1.414
• With π: π ≈ 3.1416

21. Symbol: ‘°’
Name: Degree
Why it is Used: The degree symbol is used to represent angles or temperatures. In geometry, it indicates the size of an angle in degrees, while in science, it’s used for temperature in degrees Celsius or Fahrenheit.
Example:

• Angle: 90° is a right angle.
• Temperature: Water boils at 100°C 

22. Symbol: ‘⊕’
Name: Direct Sum
Why it is Used: The direct sum symbol is used primarily in linear algebra and abstract algebra to denote the direct sum of two or more vector spaces, modules, or groups. It signifies that the combined structure retains certain properties of the individual parts.
Example:

• Direct sum of vector spaces: V⊕W

23. Symbol: ‘∧’
Name: Logical AND
Why it is Used: The logical AND symbol is used in mathematical logic and set theory to represent a conjunction where both operands must be true for the entire expression to be true. It is commonly used in Boolean logic.
Example:

• Boolean logic: p∧q is true only if both p and q are true.

24. Symbol: ‘∨’
Name: Logical OR
Why it is Used: The logical OR symbol is used to indicate a disjunction, meaning that if either of the operands is true, the entire expression is true. It is widely used in logic, set theory, and computing.
Example:

• Boolean logic: p∨q is true if either p or q is true (or both).

25. Symbol: ‘∩’
Name: Intersection
Why it is Used: The intersection symbol is used in set theory to represent the common elements between two or more sets. It shows the elements that belong to all sets in question.
Example:

• A∩B represents the elements common to both sets A and B.

26. Symbol: ‘∪’
Name: Union
Why it is Used: The union symbol represents the combination of two or more sets, including all elements from each set, without duplication. It is used in set theory and probability.
Example:

• A∪B includes all elements from both sets A and B.

27. Symbol: ‘∅’
Name: Empty Set
Why it is Used: The empty set symbol is used to denote a set with no elements. It is a fundamental concept in set theory, indicating that a set does not contain any members.
Example:

• A∩B = ∅ means that the two sets, A and B, have no elements in common.

28. Symbol: ‘⊂’
Name: Subset
Why it is Used: The subset symbol indicates that all elements of one set are contained within another set. It is used in set theory to show the relationship between two sets.
Example:

• A⊂B means that every element of set A is also in set B.

29. Symbol: ‘⊃’
Name: Superset
Why it is Used: The superset symbol indicates that one set contains all the elements of another set. It is the inverse of the subset symbol and is used in set theory.
Example:

• B⊃A means that set B contains every element of set A.

30. Symbol: ‘⇒’
Name: Implies
Why it is Used: The implies symbol is used in logic to indicate that one statement logically follows from another. If the first statement is true, then the second statement must also be true.
Example:

• If p⇒q, then whenever p is true, q must also be true.

Uncommon (Less Used) Symbols in Mathematics

31. Symbol: ‘∇’
Name: Nabla (Gradient)
Why it is Used: The nabla symbol is used in vector calculus to denote the gradient of a scalar field or the divergence or curl of a vector field. It is an essential operator in multivariable calculus and physics.
Example:

• Gradient: ∇f (x,y,z) represents the vector of partial derivatives of a scalar field f.

32. Symbol: ‘ℵ’
Name: Aleph (Cardinality of Infinite Sets)
Why it is Used: The aleph symbol is used in set theory to represent the cardinality (size) of infinite sets. The smallest infinite cardinality is denoted as ℵ0ℵ_0ℵ0, which corresponds to the cardinality of the set of natural numbers.
Example:

• The cardinality of the set of integers is ℵ₀.

33. Symbol: ‘⊗’
Name: Tensor Product
Why it is Used: The tensor product symbol is used in linear algebra and quantum mechanics to represent the tensor product of two vector spaces, matrices, or other algebraic structures. It is used to build higher-dimensional spaces.
Example:

• Tensor product: A⊗B represents the tensor product of two matrices or vectors, A and B.

34. Symbol: ‘∘’
Name: Function Composition
Why it is Used: The function composition symbol is used to indicate that one function is applied to the result of another function. It is commonly used in algebra, calculus, and computer science.
Example:

• (f∘g) (x)=f (g (x)) means that the function g(x) is applied first, followed by f(x).

35. Symbol: ‘ℜ’
Name: Real Part
Why it is Used: The real part symbol is used to denote the real part of a complex number. In complex analysis, any complex number z=a+biz = a + biz=a+bi has a real part aaa.
Example:

• For z=3+4i, ℜ(z)=3

36. Symbol: ‘ℑ’
Name: Imaginary Part
Why it is Used: The imaginary part symbol is used to represent the imaginary component of a complex number. For a complex number z=a+biz = a + biz=a+bi, the imaginary part is bbb.
Example:

• For z = 3+4i, ℑ(z) = 4

37. Symbol: ‘Δ’
Name: Delta (Change)
Why it is Used: The delta symbol is used to represent a change or difference in values. It is commonly used in calculus, physics, and other scientific fields to denote a variation in a variable.
Example:

• In calculus, Δx represents the change in x.

38. Symbol: ‘∝’
Name: Proportional To
Why it is Used: The proportional to symbol is used to indicate that two variables are proportional to each other, meaning they increase or decrease at the same rate.
Example:

• If y∝x, the y = kx, where k is a constant.

39. Symbol: ‘∈’
Name: Element Of
Why it is Used: The element of symbol is used in set theory to show that an object is a set member. It is commonly used in discussions about sets and their elements.
Example:

• x ∈ A means that x is an element of set A.

40. Symbol: ‘∉’
Name: Not Element Of
Why it is Used: The element of the symbol is used in set theory to denote that an object is not a set member.
Example:

• x ∉ B means that x is not an element of set B.

Conclusion

Mastering mathematical symbols is essential for anyone working with numbers, equations, or logic.

These 40 symbols, ranging from everyday use in basic operations to more specialized functions in higher-level math, provide just a glimpse of the depth and versatility of mathematical notation.

Understanding these symbols improves problem-solving skills and opens doors to advanced concepts in various disciplines, such as engineering, physics, and computer science.

Whether preparing for an exam, solving real-world problems, or exploring complex theories, this comprehensive guide equips you with the tools to navigate mathematics confidently.

Bookmark this list as a reference, and revisit it whenever you need clarity on mathematical symbols and their practical applications. With this knowledge, you’ll approach math with greater ease and precision.